Tutorial Review of Synthetic-aperture Radar (Sar) With Applications to Imaging of the Ocean Surface

An electromagnetic moving ridge is a self-propagating wave (requiring no medium to travel through) consisting of electric and magnetic fields whose intensities follow a sinusoidal design. These fields are perpendicular to each other and in stage as shown in Figure two.

One wavelength () is the distance from one crest to the next crest. The frequency (f) of the wave is the number of crests to travel one wavelength per unit time. The propagation velocity (or stage velocity) of the moving ridge is dependent on the medium through which it is traveling. It is calculated as:

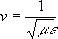
where and are the permeability and permittivity, respectively, of the medium. These are inherent electromagnetic qualities specific to unlike types of matter. The lowest values these can have are ₀ and ₀ which occur for a vacuum (free space). Thus the maximum velocity of light is inside a vacuum and is equal to:

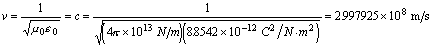
Air is and then diffuse that within information technology the velocity of an EM wave can still be considered to exist c. The wavelength and frequency are related to the velocity by the equation:

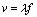
where v is the velocity in metres per second, f is the frequency in Hertz (cycles per 2d) and is the wavelength in metres.

The ratio of the electric field intensity E to the magnetic field intensity B at any moment is e'er equal to the speed of light in a vacuum.

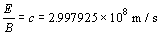
The polarization of the EM wave refers to the direction of the electric field vector. In radar scientific discipline, the wave is said to be polarized in the management of its East vector.

Principles of Real Aperture Radar

The two main types of radar images are the circularly scanning plan-position indicator (PPI) images and the side-looking images. The PPI applications are limited to the monitoring of air and naval traffic. Remote sensing applications utilise the side-looking images which are divided into two types--real discontinuity radar (unremarkably called SLAR for side-looking airborne radar or SLR for side-looking radar) and constructed aperture radar (SAR).

The SLAR imaging system uses a long, straight antenna mounted on a platform (aircraft or satellite), with its longitudinal axis parallel to the flight path. The antenna emits pulses of electromagnetic energy directed perpendicular to the flight path of the platform and downwardly to the surface of the World, as illustrated in Effigy 3. These pulses autumn within a narrow surface area on the basis and are scattered, usually in many directions, including the direction of the antenna. The return echoes get in at the antenna at different times, depending on the altitude from the antenna to the specific scattering object on the ground.

Range Resolution

Range is the direction perpendicular to flying path of the aircraft. The vertical beamwidth , shown in Effigy 3, is determined by the wavelength () and the antenna height W _a such that _five = / Westward _a . Referring to Figure iv, we come across that the width of the basis swath W _g , also chosen the range extent, is simply calculated as: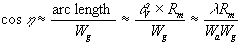

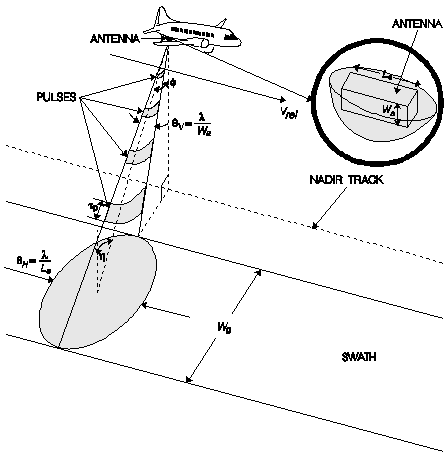
Effigy iii. Geometry of a side-looking real aperture radar.

so

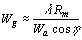
where is the incidence angle of the beam at the midpoint of the swath (equal to the look angle ) and R _yard is the slant range (distance) from the antenna to the midpoint of the swath.

Figure 4. Range resolution of a side-looking real aperture radar.

Basis resolution is defined as the minimum distance on the ground at which two object points can exist imaged separately, shown in Effigy iv every bit the distance 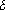R _{one thousand} . Two objects can be distinguished if the leading edge of the pulse echo from the more distant object arrives at the antenna afterward than the trailing edge of the pulse echo from the nearer object. Thus if their distances to the antenna are different by at to the lowest degree half of the pulse length (the distance the pulse travels in the time _p during which the transmitter is turned on) then the objects are resolvable. Therefore the footing range resolution is

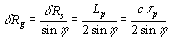
where 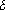R _yard is the slant range resolution, is the incidence bending, L _p is the pulse length (in units of distance), _p is the pulse duration (in units of fourth dimension) and c is the speed of light. Note that as you approach the nadir line direct below the aircraft, approaches nix making sin() approach zero so that the ground range resolution becomes extremely poor. This makes sense since the objects near the nadir line are all most the same distance from the antenna, making them impossible to distinguish.

To attain a ground range resolution modest enough to be useful, the pulse elapsing �p would take to be too short to generate enough energy to produce a sufficient echo bespeak to noise ratio (SNR). To become around this, a technique known as pulse pinch is oft used together with a type of processing of the returned signal known as matched filtering (which nosotros will not go into here, though a good description can be found in Curlander, encounter SAR References) to produce both loftier resolution and a high SNR. The ground range resolution possible with these techniques is

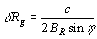
where B _R is the frequency bandwidth of the transmitted radar pulse. The resolution can exist further improved by increasing the frequency bandwidth.

Azimuth Resolution

Azimuth is the direction parallel to the flying path of the shipping. Figure 3 shows that the angular spread of the radar beam in the azimuth direction is equal to _H = / L _a where is the wavelength of the transmitted beam and L _a is the length of the radar antenna in the azimuth direction. This spread is due to interference of the waves emitted from and received by the dipoles of the antenna, which causes the angular spread to subtract as the aperture length increases (a longer antenna produces a tighter beam, much as a longer butt of a gun produces less dispersion). 2 objects on the ground and with the aforementioned slant range R tin can but be imaged separately if they are not both inside the radar beam at the aforementioned time. Thus the azimuth resolution is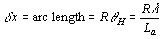

Principles of Constructed Discontinuity Radar

Considering of the way in which microwaves interact with the temper and the ground, but a select few frequency bands are useful for imaging. These are shown in Figure 5. The wavelength affects the penetration depth and also the size of a target necessary to return the radar. This shall be discussed later on.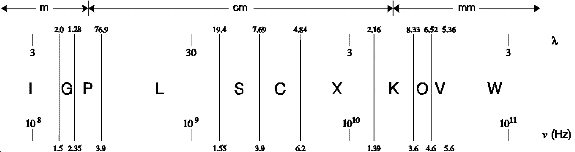

Figure five. Microwave frequency bands useful for imaging.

For a specific frequency f (or wavelength ) and slant range R, the azimuth resolution is entirely dependent on the aperture length L _a . However, for the altitudes at which satellite or airborne imaging sensors operate (in the ane-10 GHz region), technology difficulties make it is impossible to achieve values of L _a / greater than several hundred. For instance, the new Canadian satellite RADARSAT, launched in 1995, orbits at 792 km and operates at a frequency of 5.three GHz (C-Band). The real aperture limit to the azimuth resolution would be

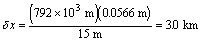
Through the employ of synthetic aperture applied science, nonetheless, RADARSAT tin can achieve a resolution equally low as 9 m.

Information technology was in 1951 that Carl Wiley beginning realized that the Doppler spread of the echo signal could be used to synthesize a much longer aperture (thus the proper name synthetic discontinuity radar) to profoundly ameliorate the resolution of a side-looking radar.

The Doppler Effect

When a detector moves with respect to a source of waves, a shift in frequency is observed known every bit the Doppler result. When a source emitting waves with frequency f recedes from the observer at a velocity v, the observed frequency is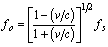

When the source approaches the observer, the observed frequency is

where v is always positive. Thus a receding source exhibits a lower frequency and an approaching source exhibits a higher frequency than the actual frequency emitted.

When 2 target points on the ground are separated in the azimuth management, they are at slightly different angles from the antenna with respect to the line of flight. Because of this, they have slightly different speeds at any given moment relative to the antenna. Therefore the signal echoed from each target will take its frequency shifted a different amount from the original. To calculate the Doppler frequency shift for a specific target, refer to Figure half-dozen as we first calculate dR/dt--the relative speed with which the target and antenna approach each other.

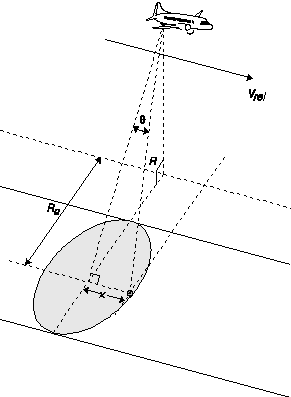
Effigy 6. SAR geometry for calculating the Doppler frequency shift for a betoken target.

To discover the R-directional component of V _rel (the velocity with which the target approaches the sensor), we can project V _rel (parallel to 10) onto a line parallel to R to become

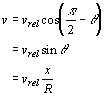
Now, nosotros can calculate the frequency observed by the ground target:

But for five << c, v ² / c ^two approaches 0. Therefore

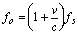
This frequency is different from the actual frequency emitted from the antenna by the corporeality

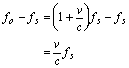
Since the return echo volition exist shifted by the same amount, the Doppler frequency shift for the target is

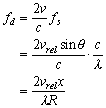
This value provides a ways of determining exactly where the repeat signal came from. For a return signal detected by the antenna at a time corresponding to the slant range R (t = twoR/c) and with a Doppler frequency shift of f _d , the azimuth coordinate must be

.

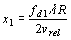Fifty-fifty if another target is at range R and within the axle at the same time, measurement of its Doppler frequency shift f _d withal allows us to associate information technology with an azimuth coordinate x ₂ . Thus for whatever target point, we now have two coordinates to localize information technology--the ground range R _g , relative to the nadir line, and the azimuth distance x _two relative to broadside.

SAR Azimuth Resolution

Utilizing the Doppler effect cannot increase the range resolution simply it does greatly increase the azimuth resolution. From the equation for Doppler frequency shift, the azimuth resolution tin can be calculated equally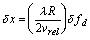

where 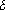f is the resolution of the Doppler frequency shift--approximately equal to the inverse of the time during which the point target was in the axle, 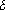f _d 1 / t _span . This fourth dimension span can be calculated equally

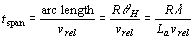
Therefore the azimuth resolution is

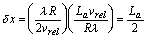
which implies that better resolution is obtained with a smaller antenna--not what 1 would expect since this is reverse to the dominion for real discontinuity radar. This does not mean, of grade, that i could simply build an antenna i cm long to obtain a resolution of 5 mm. The length of the discontinuity must be large enough to create the proper interference pattern between the dipoles of the antenna necessary for the desired beamspread at a particular frequency-- _H = / L _a .

This outcome of _x = L _a / 2 is non strictly true, however, since we assumed that a constant Doppler frequency shift was observed during the unabridged time t _bridge . In fact, f _d changes throughout the observation and is only approximately constant for a time much less than t _span . A Fourier analysis of the Doppler waveform results in frequency components at various frequencies so that the target is observed to spread out over multiple resolution cells, covering a distance greater than the resolution 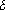x calculated above.

SAR Azimuth Compression

Every bit the radar beam passes over the indicate target, as in Figure 7, the camber range to the target varies--decreasing to a minimum so increasing again. Thus ane target traces a hyperbolic line in the azimuth-camber range aeroplane. This hyperbola is known as the range migration curve and the line joining the 2 ends is known as the rangewalk. Since this hyperbola is representative of merely 1 betoken, all the points forth this curve must be compressed to class a single pixel in the terminal prototype. There are several bug involved in this, even so. First, the shape of the hyperbolic line depends profoundly on the slant range distance to the target when at the centre of the radar beam. Second, the system impulse response changes with slant range. That is, the received point is sensitive to the time delay, thus requiring different filter parameters to maintain the constant ability requirement.

An airborne SAR has an distance depression enough to consider the Earth equally a flat surface. The hyperbola volition be very apartment since the range differences for i target are relatively small. When operating from a satellite, however, the altitude is loftier enough that the curvature of the Earth becomes a cistron, extending the hyperbola. Likewise, the rotation of the Earth warps information technology to i side in the range direction. These effects must be corrected earlier the compression processing tin begin.

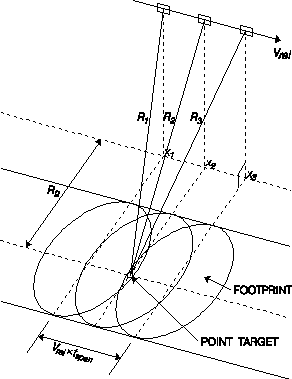
Figure 7. Hyperbolic curve traced by a point target in the azimuth-camber range aeroplane.

When ii waves are not synchronous with each other, they are said to be out of phase. The phase deviation is measured in radians or degrees. For instance, if the wave A'southward crest occurs at the aforementioned fourth dimension every bit wave B's trough, the phase difference is (or 180�). Figure 8 illustrates further examples.

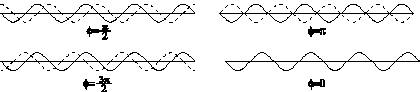
Figure 8. Phase differences between ii waves.

The phase departure, in radians, between the transmitted and received signal will exist

where Figure ix, shows that the range is

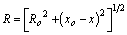
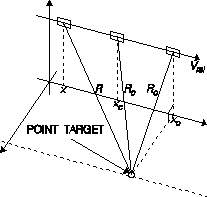
Effigy 9. Quadratic relation between range and time for a target within the radar beam.

Using the Taylor series to aggrandize this around the position x _c for the camber range R _c gives

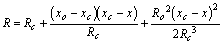
when truncated to the 2nd order. Bold that R _c and R ₀ are approximately equal for narrow beam radars, the Doppler frequency shift is

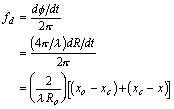
Past bold that the Doppler frequency shift is constant simply until the quadratic term adds a value of / 4 to , then the window for observing the waveform is bars to a distance of ten _window , where

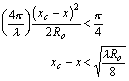
and so

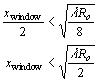
Therefore the time window is

Therefore, the new azimuth resolution is

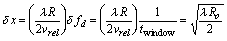
A SAR processor which uses this technique is known as unfocused SAR. This technique does not business relationship for the variable rate of phase change, but all the same manages to produce a resolution much smaller than that for real discontinuity radars. For example, using the above equation, the resolution for RADARSAT would be

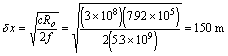
as opposed to the real aperture limit of 3.0 km.

The resolution can get fifty-fifty better, however, past using a focused SAR processor which accounts for the nonlinear stage alter. This technique uses all the data collected during the time that the target is inside the axle. The quadratic stage is adjusted such that all the render signals due to the target at ten ₀ (see Figure ix) are added coherently. Whatsoever returns from targets not at x ₀ will non hold with the adjusted returns so they will abolish. Thus the returns from the target at x ₀ will dominate the returns from other targets with the same range but not at x ₀ . With a few assumptions, this technique results in an azimuth resolution budgeted L _a / 2, which is what we calculated earlier, bold a constant phase.

Geometric Distortions

At offset glance, a SAR prototype may seem to closely resemble an optical image. Closer inspection, however, reveals striking differences which tin be used, given cognition of how radar interacts with ground features, to provide a wide spectrum of information about the targets. The most obvious deviation from an optical paradigm is the geometric distortion produced due to the low angle and the elevation or gradient of the target.

Slant vs. Ground Range

When terrain is imaged using an optical sensor such as a camera, the image can be thought of as a cardinal perspective projection--all of the terrain points accept projection lines passing through a primal point on their manner to their respective image points, as in Figure 10a. The situation for a SAR is very much different, however, in that basis objects volition be placed into locations on the image line as a function of their distance from the antenna--not equally a function of their altitude from each other on the ground. Figure 10b shows that the projection lines are concentric circles around the antenna, due to the spherical spreading of EM pulses.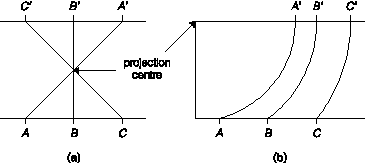

Figure 10. Image projections for (a) optical and (b) SAR imaging systems.

Suppose you are imaging objects A and B, separated a distance on the footing, as shown in Figure eleven. Because the SAR is viewing from an angle, the two points will look closer together than they actually are. Since bending ACB is approximately ninety�, we tin can say that D _g in slant range presentation is

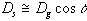
so that the distance in slant range presentation is always less than in the corresponding basis range projection. As the depression angle gets larger, D _s gets smaller for a given distance D _thousand . Thus the camber range presentation compresses the terrain features in the near range more than in the far range. This has the effect of greatly stretching the ground range project near the nadir where approaches ninety�.

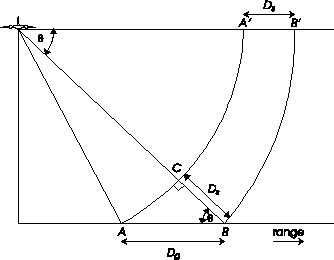
Figure 11. Image of points A and B in slant range and ground range presentations.

The ground range presentation is used when ane wishes to match the paradigm with known target locations (i.eastward. geocoding the image) on the ground. EarthView is able to convert an image from slant range to ground range and visa versa, taking into account the curvature of the Earth for satellite imagery.

Relief Displacement

Imaging a target on peak of a vertical structure height h above the ground results in a relief displacement, that is, the target is displaced a distance of r _southward in the original slant range presentation of the paradigm, equally shown in Figure 12.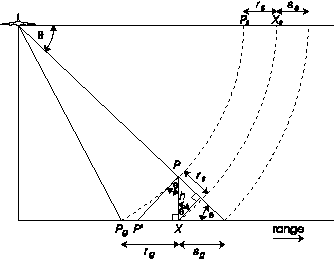

Figure 12. Relief displacement due to a vertical structure.

The relief displacement r _yard is equal to the altitude 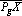, nevertheless for a small enough value of h, we run into that P _m X P'X. Thus we can estimate the relief displacement in ground presentation as

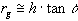
and in slant range presentation every bit

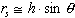
Thus the relief displacement is proportional to the height of the vertical structure and increases the closer that structure is to the nadir line.

Lay Over

The direction of relief deportation is different for optical and radar systems. A camera sees the relief displaced away from the nadir point while the SAR run across the relief displaced towards the nadir line. This is illustrated in Figure 13.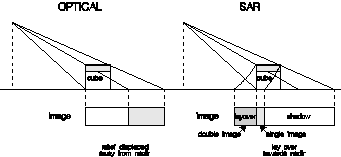

Effigy xiii. A comparison of relief displacement for (a) optical and (b) SAR systems.

Shadows

The other outcome of vertical structures is to produce shadows in the SAR image. Figures 12 and 13 illustrate this effect quite well. Considering the radar axle is at an bending, there will be a region of ground backside the vertical structure that the beam cannot accomplish. Thus for the time menstruum corresponding to that ground surface area, no echoes will be returned. This results in a black surface area on the image which is called a shadow. From Figure 12, the length of the shadow is calculated equally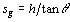

in basis range presentation and

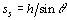
in slant range presentation. Thus the length of the shadow is proportional to the height of the vertical structure and increases the farther abroad that structure is from the nadir line or the lower the SAR antenna is.

Image of a Slope

The furnishings of imaging sloping terrain with radar are illustrated in Figure 14. Due to the vertical height, relief displacement, lay over and shadows tin effect, depending on the steepness of the slope. Slopes facing the nadir line can showroom the effects of (a) lay over, (c) foreshortening and the in-between case (b) for which the front slope appears but equally a line. Case (b) just occurs when the gradient is parallel to the nadir line and the peak angle equals the slope angle exactly.

Slopes facing away from the nadir line can exist (a) fully imaged, (c) subconscious in the radar shadow or (b) strangely imaged equally the radar beam just skims along the surface. For instance (b), slight irregularities on the slope's surface would have magnified effects. For instance, a bump nearly the summit would block the radar from the remainder of the slope causing a shadow to appear in the image.

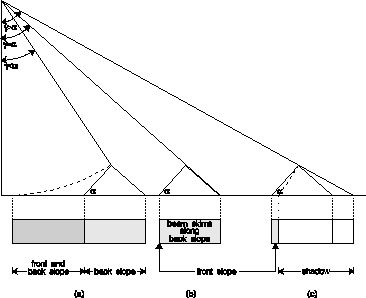
Figure xiv. Image furnishings produced by sloping terrain.

All of these slope effects can be found in Figure 15--a subsection of the SAR paradigm 82LWERS1 ( ESA) from the ERS-ane satellite, provided with EarthView.

Figure 15. ERS-one SAR image illustrating the effects of uneven terrain ( ESA).

Surface Interaction with the Radar Beam

When an electromagnetic moving ridge reflects off a surface, three basic properties of the surface come into play--the dielectric constant (or permittivity ), the roughness (rms summit relative to a polish surface) and the local slope.

Assuming that the EM moving ridge is traveling through a medium that is homogeneous (properties practice not vary from point to indicate), isotropic (properties are the aforementioned in all directions from any given betoken) and non-magnetic, then from Maxwell's equations we can express the complex electrical field vector as

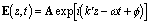
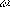where A is the amplitude vector, z is the distance traveled in time t and is a stage offset. The angular frequency is

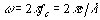
where f _c is the carrier frequency (the frequency emitted from the antenna) and is the wavelength. The expression for k' is

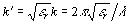
where one thousand is the wavenumber and _r is the relative permittivity (also called the dielectric constant) of the medium ( _r = / ₀ where is the permittivity of the medium and ₀ is the permittivity of costless infinite). Information technology should be noted that _r is a function of the frequency of the EM wave. The relative permeability _r has been assumed to be equal to 1 which is accurate plenty for microwave frequencies.

The polarization of the electric field East is the direction of the amplitude vector A at some instant in time. For a linearly polarized moving ridge, the direction of A is fixed in 1 plane relative to the direction of propagation. An elliptically polarized wave has an electrical field consisting of two different components oriented forth orthogonal axes such that

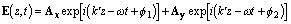
resulting in the total aamplitude vector A slowly rotating well-nigh the z-axis. If A _x = A _y and [ _ane - _ii ] = /2, then the wave becomes circularly polarized.

An EM moving ridge interacting with a surface is called handful. There are 2 types�surface scattering and volume scattering. Surface handful occurs at the interface betwixt two different homogeneous media such as the atmosphere and the Globe'south surface. Volume scattering is the effect of interaction with particles inside a not-homogeneous medium.

Surface Scattering

A smooth surface acts as a mirror, reflecting the EM wave at an bending equal and opposite to the incidence angle . Thus a shine level surface is seen as blackness in the SAR epitome since the echoes never attain the antenna. The Rayleigh criterion defines a surface as smooth if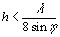

where h is the surface roughness, defined as the root-mean-square (rms) height relative to a perfectly smooth surface, is the wavelength of the wave and is the angle of incidence. Peake and Oliver (1971) modified this criterion by defining upper and lower values of h for surfaces of intermediate roughness to obtain a smoothness criterion of

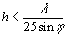
Using the Rayleigh criterion, for instance, when RADARSAT is operating at v.3 GHz with an incidence bending of fifty�, level terrain with h < 9 mm will evidence up as solid black.

Specular Handful

Any returns from a smooth level surface are due to specular scattering, in which the EM moving ridge's angle of incidence is equal and opposite to the bending of reflection and the transmission through the surface obeys Snell'south Law: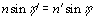

where n and n' are the alphabetize of refractions of the ii mediums, is the angle of incidence and ' is the angle of refraction, shown in Figure 16. However, the index of refraction is equal to

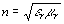
For a not-magnetic medium, _r = i, and for air, _r 1. Therefore Snell's Constabulary becomes

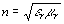
Figure sixteen. Specular scattering mechanism.

For the perfect specular reflector, radar returns (backscatter) exist only nigh vertical incidence (due to a 90� depression angle or the slope of the surface) and the reflected energy is localized to a small angular region effectually the angle of reflection. Even for not-vertical incidence, all the same, backscatter can exist for a crude subsurface if the radar is capable of penetrating deep enough. Figure xvi illustrates how it is possible to image a subsurface (i.e. the surface of a unlike medium below the top surface) that would otherwise exist invisible to optical sensors.

Bragg Scattering

For a homogeneous medium with a slightly rough surface (an rms height variation of less than /8), the scattering can exist described using the Bragg model. The surface features tin be described in terms of a spatial spectrum. Any section of that spectrum which resonates with the incident moving ridge will produce a strong backscatter. Thus, a strong return will result from surface variation patterns at wavelengths of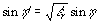

where the ascendant return is due to the wavelength 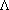for which n=one. At steep incidence angles, the backscatter is usually a combination of both specular and Bragg scattering.

The Bragg model is useful for describing the backscatter from the ocean surface. Water has a large dielectric abiding making volume scattering impossible (meet section 2.5.2). The periodic structure of ocean waves resonate well with radar waves, showing upwardly as periodic bands on the image. When imaging slightly rough terrain with little vegetation, the Bragg model tin be used to classify rock types and ages based on the surface roughness.

Book Scattering

Natural surfaces usually result in both surface scattering and volume scattering. Natural surfaces are generally inhomogeneous, pregnant they are equanimous of unlike mediums. Depending on the radar wavelength and the permittivity of the media, the EM moving ridge volition penetrate a certain depth beneath the surface. The depth at which the refracted portion of the moving ridge will be adulterate by one/e of its original power at the surface boundary is

where the relative dielectric constant is given past the circuitous number _r = ' + i '' and ''/' must be less than 0.1 for the equation to be valid.

Since every type of material has its own characteristic dielectric constant _r , the media are dielectrically variant which results in volume scattering. If the different media are mixed together in a mostly random style, the backscatter volition be in many directions. The fraction of the incident wave that is reflected back to the antenna, then, depends on the shape, density and orientation of the different media as well as on the relative permittivity between them.

Complex Dielectric Constant

The circuitous dielectric abiding of most natural materials, when dry out, is between 3 and 8 (for a typical radar frequency). For such values, the penetration depth is quite large and the reflectivity correspondingly small. The dielectric constant for water, on the other mitt, is effectually 80 (for the same frequency) resulting in high reflectivity from the surface and about no penetration. The complex dielectric constant for a material varies almost linearly with the moisture content per unit volume. The higher the moisture content, the lesser the penetration depth is and the greater the reflectivity is. The observed variation in reflectivity from natural surfaces is mainly due to the moisture content of the vegetation or soil. Thus an assay of the returns for a specific region allows for classification of the type of soil or vegetation beingness imaged.

Since the dielectric abiding depends on the frequency of the EM wave, so does the reflectivity. The college the frequency (or the lower the wavelength), the lesser the penetration is. If ane is imaging a forest, for instance, using a high frequency will result in surface scatter from the meridian of the vegetation canopy while a lower frequency would permit the EM waves to penetrate the canopy, producing volume scattering from the leaves, branches, trunks or basis.

Polarization

The polarization of an EM moving ridge refers to the orientation of the electric field intensity vector. A SAR unremarkably transmits a wave such that it is horizontally polarized. Most of the received free energy is all the same horizontally polarized, however a pocket-sized portion of information technology may be depolarized through interaction with the terrain, creating many different components at diverse angles of polarization.

Using a filter at the antenna, all polarizations tin can be screened out except for the desired polarization--either horizontal or vertical. The HH (transmit and receive horizontal) configuration is called the similar-polarized render while the HV (transmit horizontal and receive vertical) configuration is called the cross-polarized render. The HV return requires a much higher antenna proceeds than the HH due to its much smaller energy.

The images produced past these two returns may differ due to the differences between the scattering processes for each. Depolarization, for instance, is commonly explained in terms of book besprinkle or multiple reflections. At short wavelengths, when the terrain can be considered very rough, like- and cantankerous-polarized images are almost identical. At longer wavelengths, still, when the terrain is considered relatively smoothen, noticeable differences stand up out. This has resulted in express geological application in identifying rock types.

Some of the new polarimetric SARs are capable of transmitting and receiving both horizontally and vertically thus assuasive HH, HV, VH and VV returns all at the same fourth dimension. Mathematical analysis of these returns can create a geometric basis which tin can be used to synthesize the image for any possible transmit/receive polarization for the entire 360� spectrum. Certain objects, especially those man-made, have been found to stand out at a specific transmit/receive polarization, thus making their detection easier in the future.

Speckle

All SAR images inherently incorporate noise, whether due to the hardware or to stray radiations from other sources or to the phenomenon known equally speckle. Speckle manifests itself in the epitome equally the apparently random placement of pixels which are conspicuously brilliant or dark. It arises from the requirement that a SAR paradigm be formed using coherent radar--radar that tin can provide a Doppler resolution.

A unmarried resolution jail cell, or pixel, corresponds to an area of a sure size on the ground. Within that surface area, in that location exist many dissimilar targets, or scatterers, which provide the surface texture necessary for backscatter. Fifty-fifty though the surface area may exist something on the guild of 15 10 15 metres, the wavelength of the EM wave may exist only several centimetres--capable of interacting with each individual scatterer. These multiple interactions produce echoes which interfere with each other in either a constructive or destructive fashion. Constructive interference results in a strong return signal and a bright pixel in the image. Destructive interference results in a weak return signal and dark pixel in the image.

By interpreting speckle equally a random noise effect, diverse methods tin can be used to reduce speckle. Neighbouring pixels can exist averaged together to spread the abnormality out at the cost of resolution. This is known as a multiple look image. EarthView provides a median filter, in which yous can specify the size of the window of pixels to average, and a LUM Filter which uses a completely different rank-order-based technique. EarthView provides other filters for speckle reduction (Lee, Frost, MAP and Refined MAP) which smooth the data without blurring it as the median filter does.

baggettmixtiffinuel.blogspot.com

Source: https://www.geo.uzh.ch/~fpaul/sar_theory.html

Share this post